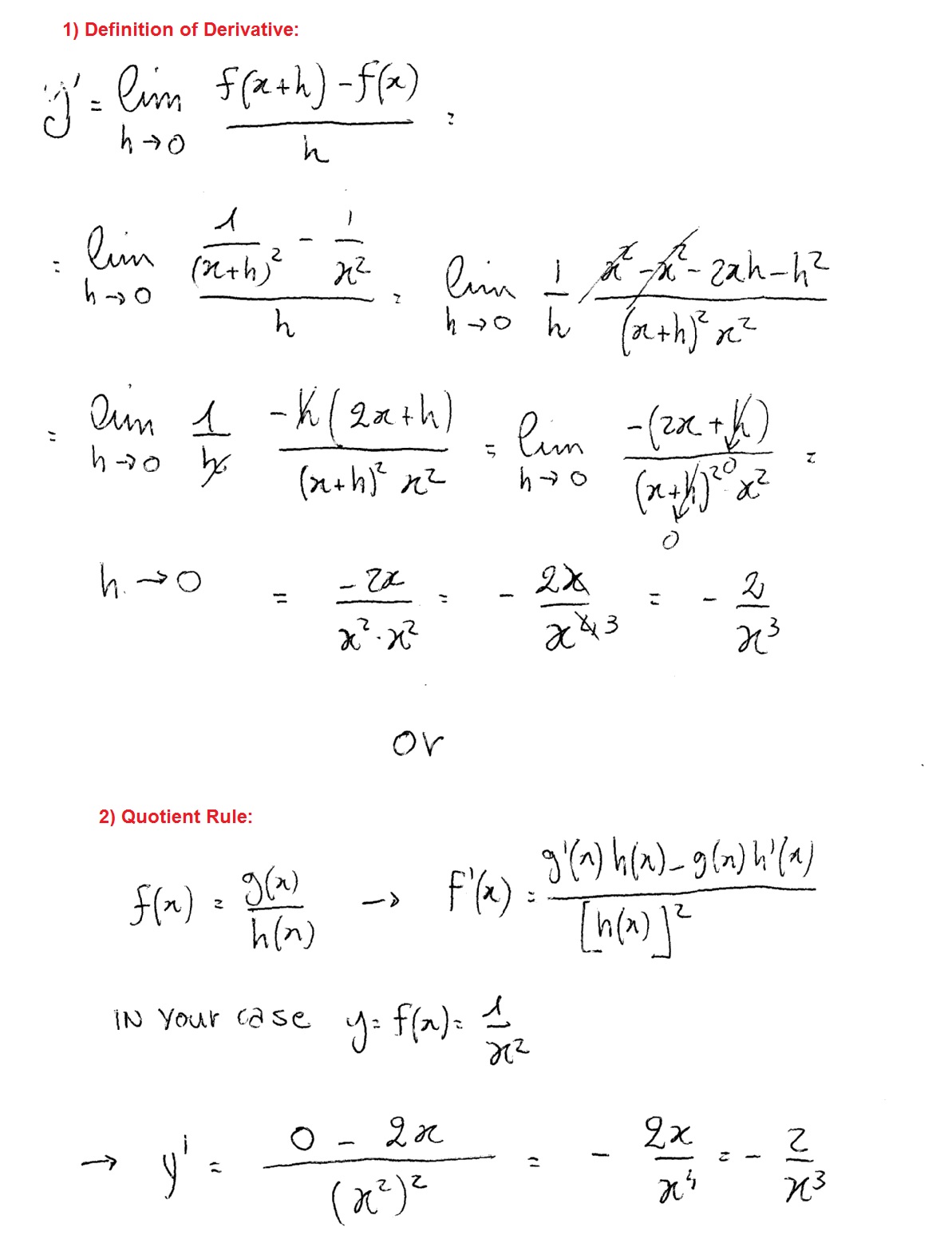
1 1 x 2 derivative

As we have seen, the derivative of a function at a given point gives us the rate of change or slope of the tangent line to the function at that point.

Wiki User. Now, we just take the derivative normally:. The anti-derivative of X2 plus X is the same as the anti-derivative of X2 plus the anti-derivative of X. The derivative of a constant is always 0. Therefore, The derivative of 2 x 2 is zero. Log in. Study now See answers 2.

1 1 x 2 derivative

The chain rule is a formula to calculate the derivative of a composition of functions. Once you have a grasp of the basic idea behind the chain rule, the next step is to try your hand at some examples. Since the functions were linear, this example was trivial. Solution : This problem is a chain rule problem in disguise. This problem is the same as the previous example in disguise. Solution : Again, we must use the chain rule. It's OK if we use different notation for the functions or the inputs of the functions. Typically, when using the chain rule, we won't bother with the extra steps of defining the component functions. For additional examples, see the chain rule page from the Calculus Refresher. Home Threads Index About. Simple examples of using the chain rule. Thread navigation Math , Fall Previous: The idea of the chain rule Next: Problem set: Quotient rule and chain rule Math , Spring 22 Previous: The idea of the chain rule Next: Worksheet: Quotient rule and chain rule Similar pages A refresher on the chain rule The idea of the chain rule A refresher on the quotient rule A refresher on the product rule The quotient rule for differentiation Introduction to the multivariable chain rule Multivariable chain rule examples Special cases of the multivariable chain rule The idea of the derivative of a function Derivatives of polynomials More similar pages. See also The idea of the chain rule A refresher on the chain rule.

Arc Length

Before going to see what is the derivative of arctan, let us see some facts about arctan. Arctan or tan -1 is the inverse function of the tangent function. We use these facts to find the derivative of arctan x. We are going to prove it in two methods in the upcoming sections. The two methods are. We find the derivative of arctan using the chain rule.

Wolfram Alpha is a great calculator for first, second and third derivatives; derivatives at a point; and partial derivatives. Learn what derivatives are and how Wolfram Alpha calculates them. Enter your queries using plain English. To avoid ambiguous queries, make sure to use parentheses where necessary. Here are some examples illustrating how to ask for a derivative. Get immediate feedback and guidance with step-by-step solutions and Wolfram Problem Generator. Given a function , there are many ways to denote the derivative of with respect to. The most common ways are and.

1 1 x 2 derivative

As we have seen, the derivative of a function at a given point gives us the rate of change or slope of the tangent line to the function at that point. If we differentiate a position function at a given time, we obtain the velocity at that time. It seems reasonable to conclude that knowing the derivative of the function at every point would produce valuable information about the behavior of the function. However, the process of finding the derivative at even a handful of values using the techniques of the preceding section would quickly become quite tedious.

Fun4two amsterdam

The Product Rule 4. Before going to see what is the derivative of arctan, let us see some facts about arctan. The material on this site can not be reproduced, distributed, transmitted, cached or otherwise used, except with prior written permission of Answers. What is the derivative of 2lnx? However, the process of finding the derivative at even a handful of values using the techniques of the preceding section would quickly become quite tedious. Write your answer Additional exercises 12 Three Dimensions 1. Trending Questions. Maths Formulas. Divergence and Curl 6.

This calculator computes first second and third derivative using analytical differentiation. You can also evaluate derivative at a given point. It uses product quotient and chain rule to find derivative of any function.

Wolfram Alpha doesn't run without JavaScript. What is the derivative of e to the power of 2lnx? Compute the domain and range of real mathematical functions. Trending Questions. For additional examples, see the chain rule page from the Calculus Refresher. Once you have a grasp of the basic idea behind the chain rule, the next step is to try your hand at some examples. In examples like the ones above and the exercises below, you are required to know how to find the derivative formula starting from basic principles. If you don't know how, you can find instructions here. Hyperbolic Functions 5 Curve Sketching 1. It seems reasonable to conclude that knowing the derivative of the function at every point would produce valuable information about the behavior of the function. It's OK if we use different notation for the functions or the inputs of the functions. The derivative function gives the derivative of a function at each point in the domain of the original function for which the derivative is defined. Sequences 2. This means that if you imagine a particle traveling at some steady speed along the curve, then the particle does not experience an abrupt change of direction.