Cos 2 2x sin 2 2x
Please ensure that your password is at least 8 characters and contains each of the following:.
We recall the Pythagorean trig identity and rearrange it for cos squared x to make [1]. We recall the double angle trig identity and rearrange it for sin squared x to make [2]. We then substitute [2] into [1] and simplify to make identity [3]. As you can see identity 3 is almost like the cos squared part of our integration problem except it has 2x for the angle. If we multiply the angles on both sides by 2, then as you can see, we get the cos squared 2x term, as shown above.
Cos 2 2x sin 2 2x
.
We recall the trig identity for cos squared 2x that we previously made, and multiply the angles by 2 on both sides again, to give the identity above.
.
Cos2x is one of the important trigonometric identities used in trigonometry to find the value of the cosine trigonometric function for double angles. It is also called a double angle identity of the cosine function. The identity of cos2x helps in representing the cosine of a compound angle 2x in terms of sine and cosine trigonometric functions, in terms of cosine function only, in terms of sine function only, and in terms of tangent function only. Cos2x identity can be derived using different trigonometric identities. Let us understand the cos2x formula in terms of different trigonometric functions and its derivation in detail in the following sections. Cos2x is an important trigonometric function that is used to find the value of the cosine function for the compound angle 2x. We can express cos2x in terms of different trigonometric functions and each of its formulas is used to simplify complex trigonometric expressions and solve integration problems. Cos2x is a double angle trigonometric function that determines the value of cos when the angle x is doubled.
Cos 2 2x sin 2 2x
Please ensure that your password is at least 8 characters and contains each of the following:. Hope that helps! You'll be able to enter math problems once our session is over. New Messages.
Design border drawing
List the new angles. The tangent function is negative in the second and fourth quadrants. Cancel the common factor. Take the inverse tangent of both sides of the equation to extract from inside the tangent. Consolidate the answers. We recall the Pythagorean trig identity and rearrange it for cos squared x to make [1]. To find the second solution , subtract the reference angle from to find the solution in the third quadrant. Convert from to. Integration Solutions Donate. We recall the trig identity for cos squared 2x that we previously made, and multiply the angles by 2 on both sides again, to give the identity above. We recall the double angle trig identity and rearrange it for sin squared x to make [2]. Write each expression with a common denominator of , by multiplying each by an appropriate factor of.
Please ensure that your password is at least 8 characters and contains each of the following:. Enter a problem
Divide by. Multiply the numerator by the reciprocal of the denominator. The tangent function is negative in the second and fourth quadrants. The period of the function can be calculated using. We recall the Pythagorean trig identity and rearrange it for cos squared x to make [1]. Simplify the numerator. The distance between and is. We integrate the second term and get the answer as shown above in red. Cancel the common factor. As you can see identity 3 is almost like the cos squared part of our integration problem except it has 2x for the angle. Add to every negative angle to get positive angles. The period of the function is so values will repeat every radians in both directions. Integration Solutions Donate.

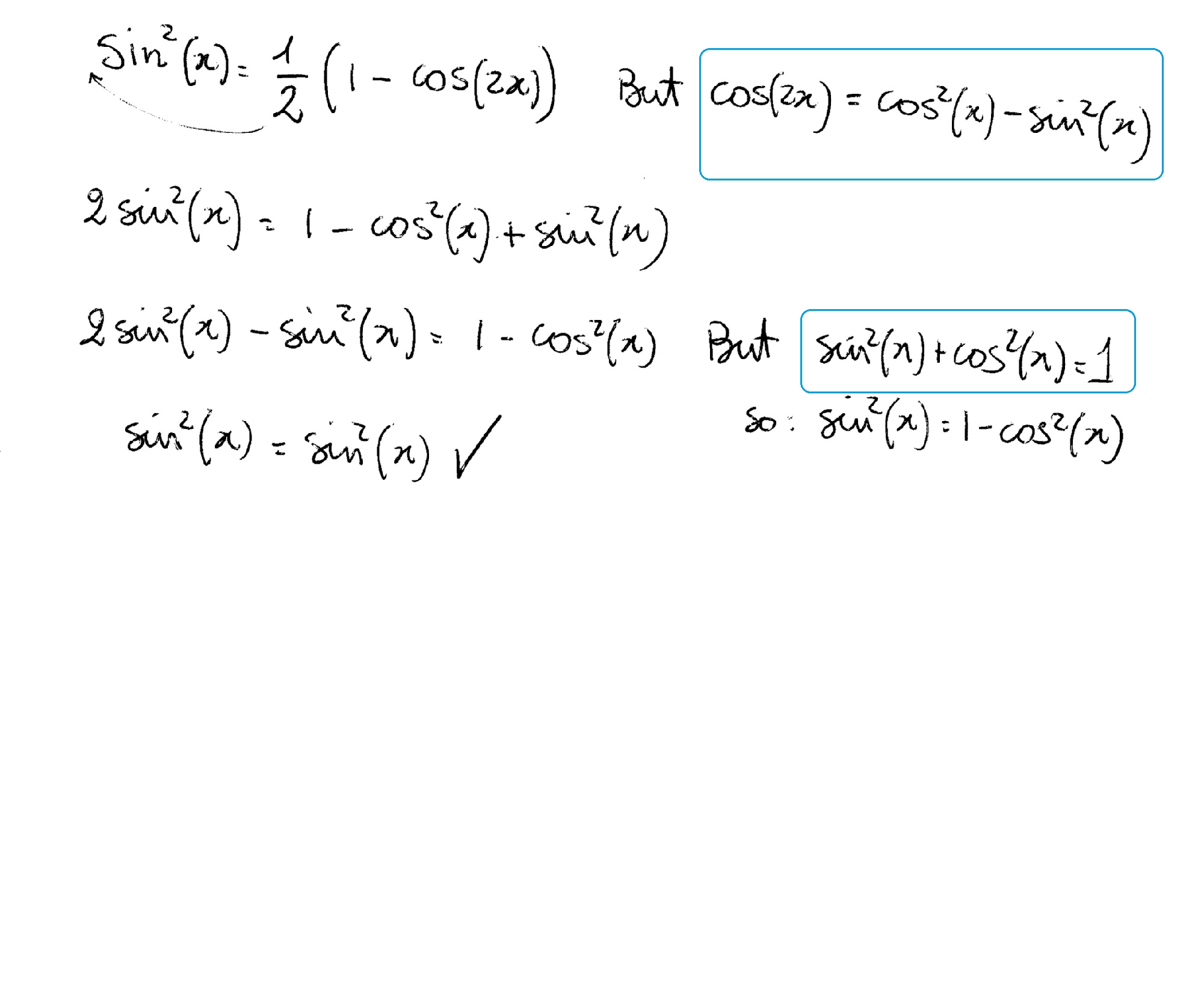
I am sorry, that I interfere, but you could not give little bit more information.
The charming message