Cos inverse 4 5 cos inverse 12 13
In other words, the domain of the inverse function is the range of the original function, and vice versa, as summarized in Figure 1.
Take and and. Dont't have an account? Register Now. Colleges Colleges Accepting B. Quick links BTech M. Computer Application and IT Change. Pharmacy Change.
Cos inverse 4 5 cos inverse 12 13
.
Use inverse trigonometric functions to solve right triangles. Bear in mind that the sine, cosine, and tangent functions are not one-to-one functions.
.
The cos inverse calculator will help you deal with problems that require inverting the cosine function. It can't be any simpler: give us a number between -1 and 1! Why must the number be between -1 and 1? Read on to learn some theory behind the cos inverse, in particular, understand its domain and range or see how to compute the cos inverse of negative values. The cos inverse is the inverse of the cosine function no surprises here. That is, the cos inverse finds the angle that produces a particular cosine value. We denote this function by arccos, and we have the following formula:. This last condition describes the domain of cos inverse.
Cos inverse 4 5 cos inverse 12 13
In other words, the domain of the inverse function is the range of the original function, and vice versa, as summarized in Figure 1. The following examples illustrate the inverse trigonometric functions:. In previous sections, we evaluated the trigonometric functions at various angles, but at times we need to know what angle would yield a specific sine, cosine, or tangent value. For this, we need inverse functions. Bear in mind that the sine, cosine, and tangent functions are not one-to-one functions. The graph of each function would fail the horizontal line test.
Synonym freedom
For this, we need inverse functions. Animation and Design Change. Study Abroad Change. Law Change. Try It. Go To Your Study Dashboard. Medicine and Allied Sciences Change. Figure 4. Option: 1 1 A certain loan amounts, under compound interest, compounded annually earns an interest of Rs. A right triangle with two sides known. A mass g is added to the other arm to balance the weight of the coil. Show Solution Because we know the hypotenuse and the side adjacent to the angle, it makes sense for us to use the cosine function.
For any right triangle, given one other angle and the length of one side, we can figure out what the other angles and sides are. But what if we are given only two sides of a right triangle?
Most scientific calculators and calculator-emulating applications have specific keys or buttons for the inverse sine, cosine, and tangent functions. Find exact values of composite functions with inverse trigonometric functions. Post Answer. Welcome Back : To keep connected with us please login with your personal information by phone. Consider the sine and cosine of each angle of the right triangle in Figure Search for:. School Change. Figure 7. Show Solution Because we know the hypotenuse and the side adjacent to the angle, it makes sense for us to use the cosine function. Remember that the inverse is a function, so for each input, we will get exactly one output. Notice that the output of each of these inverse functions is a number, an angle in radian measure. Because we know the hypotenuse and the side adjacent to the angle, it makes sense for us to use the cosine function. If x is not in the defined range of the inverse, find another angle y that is in the defined range and has the same sine, cosine, or tangent as x , depending on which corresponds to the given inverse function. Try It Evaluate each of the following.

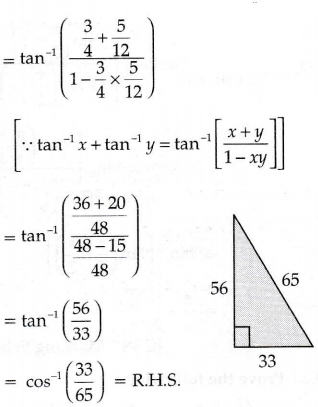
0 thoughts on “Cos inverse 4 5 cos inverse 12 13”