Derive sec 2x
Note that in this post we will be looking at differentiating sec 2x which is not the same as differentiating sec 2 x. The chain rule is useful for finding the derivative of a function which could have been differentiated had it been in x, derive sec 2x, but it is in the form of another expression which could also be differentiated if it stood on its own. Derive sec 2x perform the differentiation sec 2xthe derive sec 2x rule says we must differentiate the expression as if it were just in terms of x as long as we then multiply prada pullover result by the derivative of what the expression is actually in terms of in this case the derivative of 2x. The Chain Rule: For two differentiable functions f x and g x.
The derivative of sec square x at a point gives us the slope of graph of the function sec square x at that point. Sec x is one of the important trigonometric functions. Derivative or differentiation is the rate of change of any function with respect to one of its variables. Secant is a trigonometric function , and the derivative of sec square x is denoted as two times the product of sec square x and tan x. In this maths article we will learn about the derivative of sec square x with its formula, graph and derivation.
Derive sec 2x
The first method is by using the product rule for derivatives since sec 2 x can be written as sec x. The product rule for differentiation states that the derivative of f x. The Product Rule: For two differentiable functions f x and g x. The chain rule is useful for finding the derivative of a function which could have been differentiated had it been in x, but it is in the form of another expression which could also be differentiated if it stood on its own. Although the expression sec 2 x contains no parenthesis, we can still view it as a composite function a function of a function. Now the function is in the form of x 2 , except it does not have x as the base, instead it has another function of x sec x as the base. The Chain Rule: For two differentiable functions f x and g x. Just be aware that not all of the forms below are mathematically correct. It is interesting to note that the derivative of sec 2 x is equal to the derivative of tan 2 x. View all posts by DerivativeIt. The second method is by using the chain rule for differentiation. We can write sec 2 x as sec x 2. Published by DerivativeIt.
Learn about the Derivative of Root x and the Derivative of Sin 2x. Let us learn to evaluate the derivative of sec square x using different methods of derivatives and its formula.
The derivative of sec square x is equal to twice the product of sec square x and tanx. Differentiation of a function gives the slope function of the curve of the function which can give the slope of the function at a particular point. Let us learn to evaluate the derivative of sec square x using different methods of derivatives and its formula. We will also solve different examples related to the concept for a better understanding of the concept of derivatives. Sec x is one of the important and main trigonometric functions in trigonometry.
The chain rule comes up with a way to calculate the derivative of composite functions with both the number of functions in the composition identifying the number of differentiation steps required. When you take the derivative of the derivative of a function, this is called the second derivative of that function. Though the first derivative indicates whether the function is decreasing or increasing, the second derivative indicates whether the first derivative is decreasing or increasing. To calculate the second derivative of a function, you just need to differentiate the first derivative. Since the second derivative will be the derivative of a function having the product of two terms, therefore, the product rule will be used to work out the second derivative in this case. So the substitution of these values in the above formula will give us:. The chain rule is the method used to calculate the derivative of a composite function. The chain rule only applies to composite functions. The derivative of the composition of these two functions can be expressed using the chain rule. The secant of an angle in a right-angled triangle is the measure of the hypotenuse divided by the measurement of the adjacent side.
Derive sec 2x
Learn what is the derivative of sec square x with formula. Also understand how to prove the derivative of sec 2x by three techniques. Derivatives have a wide range of applications in almost every field of engineering and science. The sec 2x derivative can be calculated by following the rules of differentiation. Or, we can directly find the sec2x differentiation by applying the first principle of differentiation. In this article, you will learn what the sec 2 x derivative is and how to calculate the sec2x derivative by using different approaches. This derivative measures the rate of change of the cos 2x function. In a right-angled triangle, sec x is the reciprocal of cos x , which represents the ratio of the hypotenuse to the adjacent side. Thus, sec x can be written as;.
Precios diesel cepsa
Published by DerivativeIt. Maths Games. Derivative of x. Secant square theta is equal to the summation of 1 and tangent square theta. Privacy Policy. The first method is by using the product rule for derivatives since sec 2 x can be written as sec x. Sec square x is an example of a composite function. What is the Derivative of Sec Square x? Download Brochure. Commercial Maths. What is sec square x?
The derivative of sec square x is equal to twice the product of sec square x and tanx. Differentiation of a function gives the slope function of the curve of the function which can give the slope of the function at a particular point. Let us learn to evaluate the derivative of sec square x using different methods of derivatives and its formula.
Sec x is one of the important and main trigonometric functions in trigonometry. Note that in this post we will be looking at differentiating sec 2x which is not the same as differentiating sec 2 x. Derivative or differentiation is the rate of change of any function with respect to one of its variables. Secant square theta is equal to the summation of 1 and tangent square theta. Derivative of Sec Square x using Product Rule Product rule in differentiation is used when we need to find the derivative of the product of two functions. Differentiating this gives us the second derivative of sec square x. On differentiating a function, we get a slope of the curve of the function at a particular point. We can use the derivative of sec x and the power rule to find the derivative of sec square x. The product rule of differentiation is used to find the derivative of the product of two functions. Skip to content The derivative of sec 2x is 2sec 2x tan 2x How to calculate the derivative of sec 2x Note that in this post we will be looking at differentiating sec 2x which is not the same as differentiating sec 2 x. Sec square x is an example of a composite function. Answer: The derivative of sec 2 x 2 w. Example 1: Determine the derivative of sec square x square with respect to x square. Learn about Differentiation and Integration. In a similar way, the derivative of sec 2x with respect to 2x is sec 2x tan 2x.

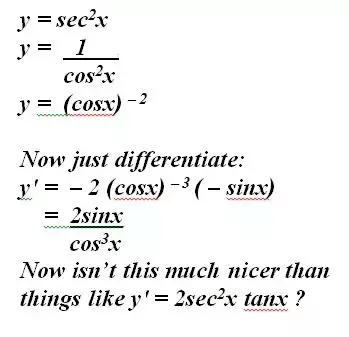
This business of your hands!
You commit an error. Let's discuss. Write to me in PM.