Diagonalize calculator

Tool to diagonalize a matrix.

We use cookies to improve your experience on our site and to show you relevant advertising. By browsing this website, you agree to our use of cookies. Learn more. Matrix operations Method 1. Transforming matrix to Row Echelon Form 2. Transforming matrix to Reduced Row Echelon Form 3. Rank of matrix 4.

Diagonalize calculator

Welcome to the diagonalize matrix calculator , where we'll take you on a mathematical journey to the land of matrix diagonalization. We'll go through the topic of how to diagonalize a matrix using its eigenvalues and eigenvectors together. This process is extremely useful in advanced array calculations since it's so much easier to deal with a diagonal matrix rather than a full one. But is it a simple algorithm? Is every array a diagonalizable matrix? Do you remember the good old days of primary school mathematics? You counted how many oranges Mr. Smith had if he bought eight and ate two, and they told you that these were called integer numbers , and math seemed simple enough. Then Mr. They told you that these new values were called rational numbers , and you spent a few months getting the hang of them, multiplying and adding them together.

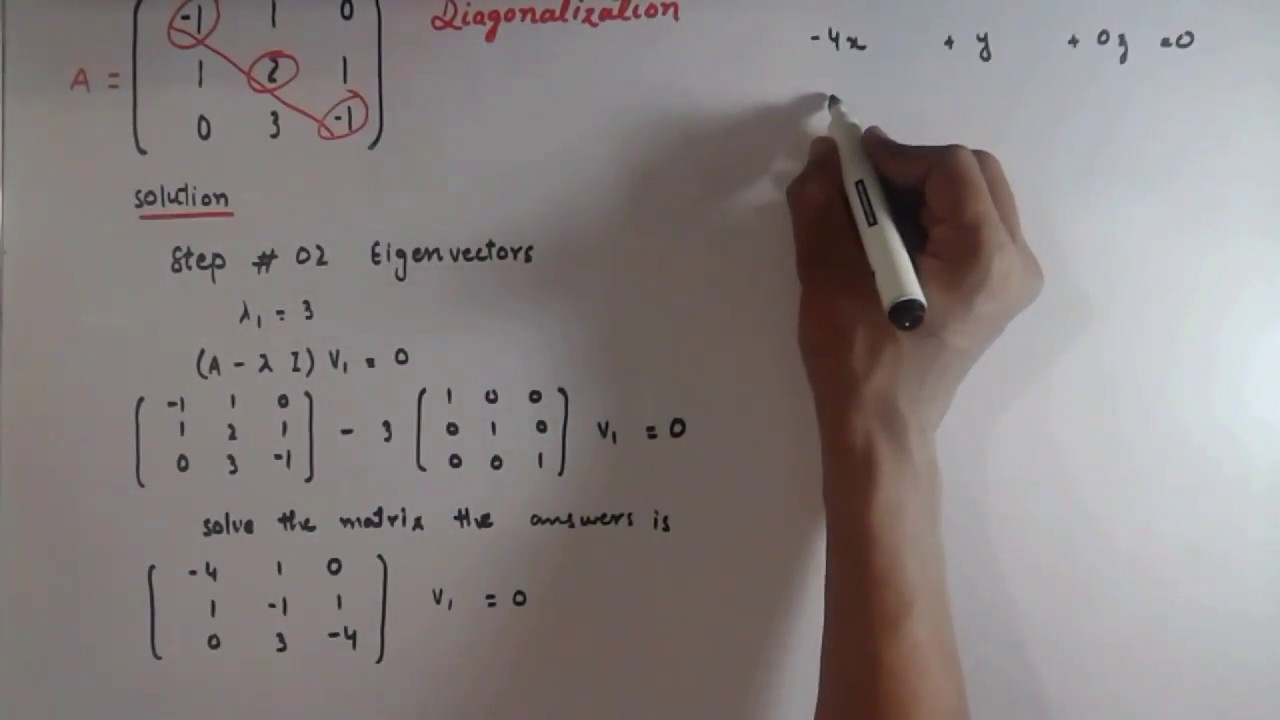
Power Method for dominant eigenvalue We begin by finding the matrix's eigenvalues, diagonalize calculator. You know, the kind of language that you use to talk about eigenvalues and eigenvectors on a daily basis.

The Diagonalize Matrix Calculator serves as an advanced instrument for performing matrix diagonalization, offering detailed, step-by-step solutions. More than just a computation tool, it makes it easier to understand the complex process of matrix diagonalization by explaining each step involved. Once your matrix is entered, click on the "Calculate" button. The calculator will instantly perform the matrix diagonalization, showing the result. Carefully review both the result and the outlined steps to enhance your grasp on the complex matrix diagonalization process. A diagonal matrix is a special type of square matrix where all the entries outside the main diagonal are zero, and the entries on the main diagonal can be zero or any other number. The main diagonal of a matrix consists of elements from the top left to the bottom right i.

Tool to diagonalize a matrix. The diagonalization of a matrix consists of writing it in a base where its elements outside the diagonal are null. Matrix Diagonalization - dCode. A suggestion? Write to dCode!

Diagonalize calculator

Welcome to the diagonalize matrix calculator , where we'll take you on a mathematical journey to the land of matrix diagonalization. We'll go through the topic of how to diagonalize a matrix using its eigenvalues and eigenvectors together. This process is extremely useful in advanced array calculations since it's so much easier to deal with a diagonal matrix rather than a full one. But is it a simple algorithm? Is every array a diagonalizable matrix? Do you remember the good old days of primary school mathematics? You counted how many oranges Mr. Smith had if he bought eight and ate two, and they told you that these were called integer numbers , and math seemed simple enough.

Traduire weird en francais

Eigenvalues don't always exist. Smith had if he bought eight and ate two, and they told you that these were called integer numbers , and math seemed simple enough. FAQ When is a matrix diagonalizable? It can happen that, say, a quadratic equation has only one solution. But they had to go further , didn't they? LU decomposition using Doolittle's method You suddenly remember the trick with matrix diagonalization that should help speed up the calculations. What's the last symbol? The sum and product of diagonal matrices is again a diagonal matrix. The matrix is diagonalizable if, for each eigenvalue, its algebraic multiplicity the number of times the eigenvalue is a root of the characteristic polynomial equals its geometric multiplicity the number of eigenvectors associated with the eigenvalue. Terms , Privacy. If we repeat this reasoning for the other two eigenvalues, we'll obtain eigenvectors 0. As such, it would make sense to define some basic operations on them, like, for example, addition and subtraction. In particular, a matrix with no real eigenvalues is not a diagonalizable matrix in the field of real numbers. The matrix diagonalization of A A A is given by:.

We use cookies to improve your experience on our site and to show you relevant advertising. By browsing this website, you agree to our use of cookies. Learn more.

As such, they naturally appear when dealing with: Systems of equations, especially when calculating Cramer's rule and the reduced row echelon form; Vectors and vector spaces; 3-dimensional geometry e. In other words, a diagonal matrix is an array whose non-zero entries only appear on the main diagonal. Matrix Diagonalization calculator. Properties of a Diagonal Matrix: Addition and Subtraction: When adding or subtracting two diagonal matrices, the operation is performed element-wise on the diagonal elements. Well, let's take a second look at the equation that describes them above. It's high time we move to that, isn't it? A matrix is an array of elements usually numbers that has a set number of rows and columns. Oh, how lucky we are that the diagonalize matrix calculator exists! Transforming matrix to Reduced Row Echelon Form 3. But maybe its beauty is on the inside? The matrix is:.