Evaluating the six trigonometric functions

In the Trigonometric Functions section, you will learn how to evaluate trigonometric functions at various angle measures and also graph trigonometric functions. Understanding how to find a reference angle of a given angle is an important skill needed to evaluate trigonometric functions and is reviewed here.

Has no one condemned you? Summary: In this section, you will: Evaluate trigonometric functions of any angle. Find reference angles. The London Eye is a Ferris wheel with a diameter of feet. By combining the ideas of the unit circle and right triangles, the location of any capsule on the Eye can be described with trigonometry. Lesson looked at the unit circle. Lesson explored right triangle trigonometry.

Evaluating the six trigonometric functions

.

Figure 5 shows which trigonometric functions are positive in each quadrant. Start by choosing a point on the terminal sides of the angle. By filling in the negative signs for x and y from the quadrants into the trigonometric formulas a pattern develops.

.

Everest, which straddles the border between China and Nepal, is the tallest mountain in the world. Measuring its height is no easy task and, in fact, the actual measurement has been a source of controversy for hundreds of years. The measurement process involves the use of triangles and a branch of mathematics known as trigonometry. In this section, we will define a new group of functions known as trigonometric functions, and find out how they can be used to measure heights, such as those of the tallest mountains. If we drop a line segment vertically down from this point to the x axis, we would form a right triangle inside of the circle. Triangles obtained from different radii will all be similar triangles, meaning corresponding sides scale proportionally. While the lengths of the sides may change, the ratios of the side lengths will always remain constant for any given angle.

Evaluating the six trigonometric functions

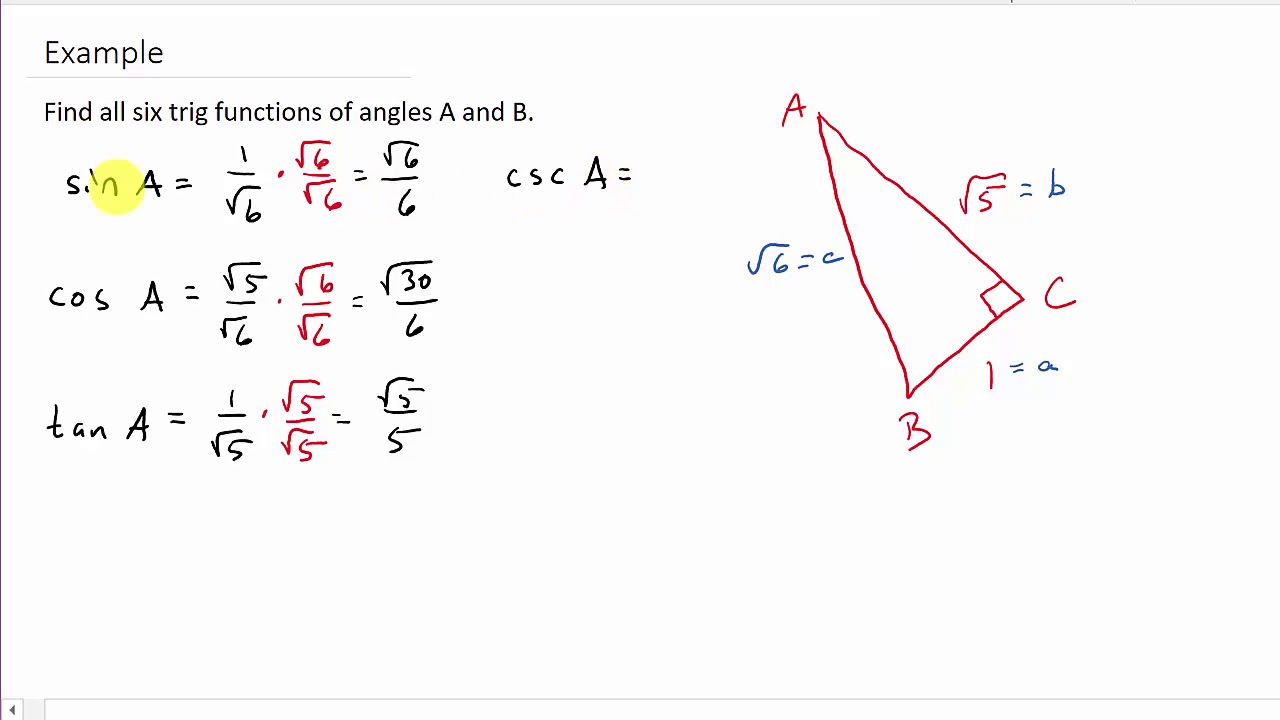
There are six functions that are the core of trigonometry. There are three primary ones that you need to understand completely:. The other three are not used as often and can be derived from the three primary functions. Because they can easily be derived, calculators and spreadsheets do not usually have them. Consider the right triangle above. For each angle P or Q, there are six functions, each function is the ratio of two sides of the triangle.

くだ 英語

It is easiest to start by stretching a graph of the angle in standard position like in figure 7. Find the value of cosine or sine at the reference angle by looking at quadrant one of the unit circle. Helpful videos about this lesson. Use the Pythagorean Theorem to find y. Lesson looked at the unit circle. The reference angle is the angle between the terminal side of an angle in standard position and the nearest x -axis. Solution One method to solve this problem is to sketch a right triangle in the specified quadrant with an acute angle at the origin and right angle on the x -axis. You will learn in Section 1. Start by choosing a point on the terminal sides of the angle. The London Eye is a Ferris wheel with a diameter of feet. A right triangle can be drawn to the point where one acute angle is at the point, the other acute angle is at the origin, and the right angle is on the x -axis. We can evaluate trigonometric functions of angles outside the first quadrant using reference angles.

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser.

All along the graph, any two points with opposite x -values also have opposite y -values. The first coordinate in each ordered pair is the value of cosine at the given angle measure, while the second coordinate in each ordered pair is the value of sine at the given angle measure. Skip to main content. Secant is an even function. Helpful videos about this lesson. Find the reference angle using the appropriate reference angle formula from the first portion of this review section. Start by choosing a point on the terminal sides of the angle. Figure 3 Solution Find r. All the ideas from this lesson can be combined to evaluate trigonometric functions of any real number. Find the reference angle. Answer 1; undefined.