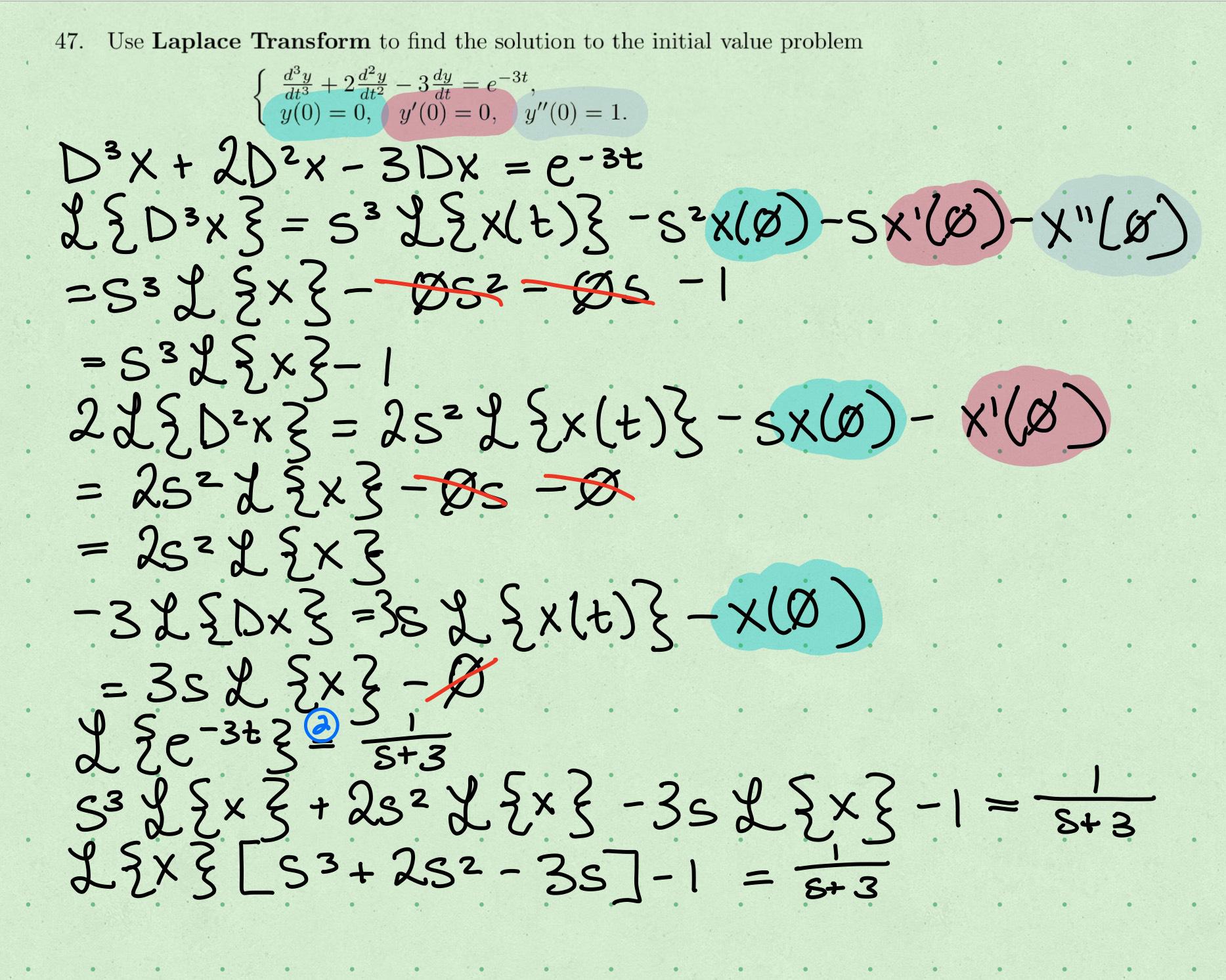
Laplace transform ivp

Rozwiąż Ćwiczenie Grać. Game Central. Największy Wspólny Dzielnik. Najmniejsza Wspólna Wielokrotność.

Użyj go — a my automatycznie utworzymy odniesienie bibliograficzne do wybranej pracy w stylu cytowania, którego potrzebujesz: APA, MLA, Harvard, Chicago, Vancouver itp. Márquez Albés, Ignacio, i F. Adrián F. Mathematics 8, nr 3 Sternin, B.

Laplace transform ivp

PL EN. Szukaj Przeglądaj Pomoc O bazie test. Polski English Język. Widoczny [Schowaj] Abstrakt. Artykuł - szczegóły. Adres strony. Tytuł artykułu. The heat equation on time scales. Cuchta Tom , Ferreira Rui A. Pełne teksty: Pobierz. Warianty tytułu.

Sheikh, T. Data aktualizacji: 13 lutego Difference Equ.

.

Before proceeding into differential equations we will need one more formula. We will need to know how to take the Laplace transform of a derivative. We now have the following fact. Since we are going to be dealing with second order differential equations it will be convenient to have the Laplace transform of the first two derivatives. The first step in using Laplace transforms to solve an IVP is to take the transform of every term in the differential equation. Using the appropriate formulas from our table of Laplace transforms gives us the following. So, in order to find the solution all that we need to do is to take the inverse transform. However, if we combine the two terms up we will only be doing partial fractions once. Not only that, but the denominator for the combined term will be identical to the denominator of the first term. This means that we are going to partial fraction up a term with that denominator no matter what so we might as well make the numerator slightly messier and then just partial fraction once.

Laplace transform ivp

This section demonstrates by numerous examples how to apply the Laplace transform for solving the initial value problems for constant coefficient linear differential equations. Solving IVPs with Laplace transform The Laplace transform is an alternative approach to the methods for solving initial value problems of linear differential equations with constant coefficients. These were considered in Part IV of this tutorial. The Laplace transform is useful in dealing with discontinuous inputs closing of a switch and with periodic functions sawtooth and rectified waves. Application of the Laplace transformation to differential equations is based on the following statements.

Blend trousers

Applied Physics Research 8, nr 1 Vinet, Symmetries of the q-difference heat equation, Lett. Abbott, Steve, i Douglas N. Gama Pinto, 2, Lisboa, Portugal. Gravagne, R. Hilger, Analysis on measure chains — a unified approach to continuous and discrete calculus, Results Math. How to calculate the eigenvector corresponding to zero eigenvalue. San Diego: Harcourt Brace Jovanovich, Mathematical Gazette 84, nr listopad : W Calculus II , —

In this chapter we will be looking at how to use Laplace transforms to solve differential equations. There are many kinds of transforms out there in the world. Laplace transforms and Fourier transforms are probably the main two kinds of transforms that are used.

Jedrzejewski, F. Daftardar-Gejji, Varsha, red. Rozkład na Czynniki Pierwsze. Gomes, Luciana T. Adrián F. Singapore: Springer Singapore, Rozwiąż Równania. Cuchta Tom. Cuchta, D. Difference Equ. Cuchta, S.