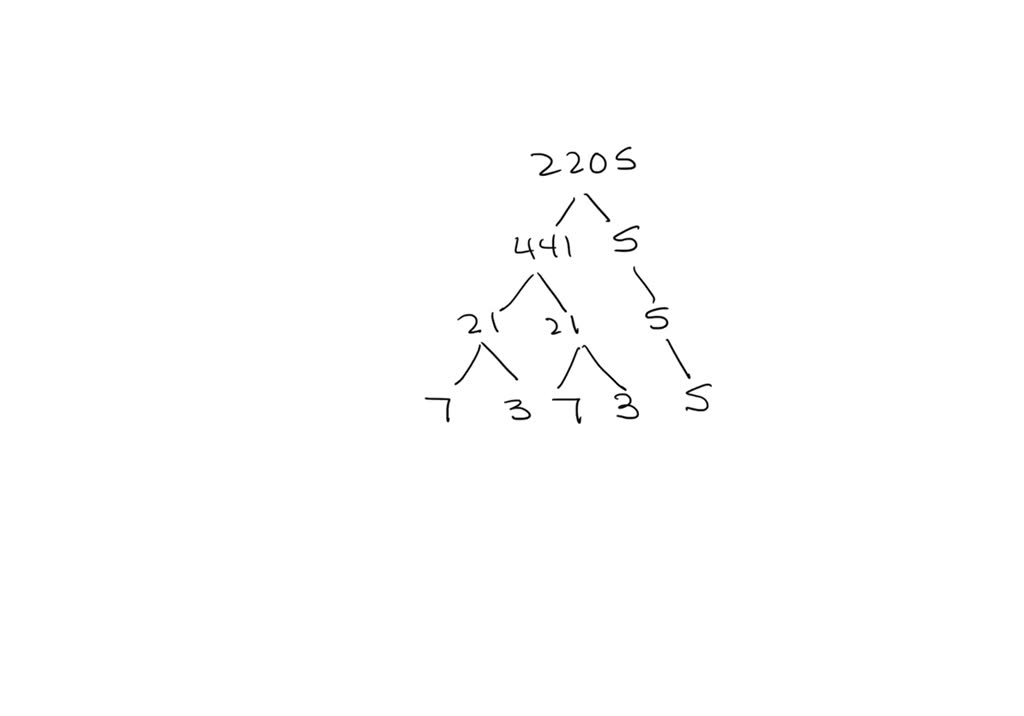
Prime factorization 2205

Factors of are numbers that, prime factorization 2205 multiplied in pairs give the product as There are overall 18 factors of among which is the biggest factor and 3, 5, 7 are its prime factors.

Wiki User. Numbers 1 to 30,! It is divisible by 3 and 5. The factors of are: 1, 3, 5, 7, 9, 15, 21, 35, 45, 49, 63, , , , , , , LCM of 45 and 49 is

Prime factorization 2205

Factors of are any integer that can be multiplied by another integer to make exactly In other words, finding the factors of is like breaking down the number into all the smaller pieces that can be used in a multiplication problem to equal There are two ways to find the factors of using factor pairs, and using prime factorization. Factor pairs of are any two numbers that, when multiplied together, equal Find the smallest prime number that is larger than 1, and is a factor of For reference, the first prime numbers to check are 2, 3, 5, 7, 11, and Repeat Steps 1 and 2, using as the new focus. In this case, 3 is the new smallest prime factor:. Remember that this new factor pair is only for the factors of , not Repeat this process until there are no longer any prime factors larger than one to divide by. At the end, you should have the full list of factor pairs. So, to list all the factors of 1, 3, 5, 7, 9, 15, 21, 35, 45, 49, 63, , , , , , ,

Multiplication Tables. Plus, learn how Thinkster can help make your child math confident for life!

.

Here is the answer to questions like: What is the prime factorization of or is a prime or a composite number? Use the Prime Factorization tool above to discover if any given number is prime or composite and in this case calculate the its prime factors. See also in this web page a Prime Factorization Chart with all primes from 1 to The prime factorization is the decomposition of a composite number into a product of prime factors that, if multiplied, recreate the original number. Factors by definition are the numbers that multiply to create another number. A prime number is an integer greater than one which is divided only by one and by itself. Note the the only "prime" factors of 72 are 2 and 3 which are prime numbers. Let's find the prime factorization of Solution 1 Start with the smallest prime number that divides into 72, in this case 2.

Prime factorization 2205

Prime numbers are natural numbers positive whole numbers that sometimes include 0 in certain definitions that are greater than 1, that cannot be formed by multiplying two smaller numbers. An example of a prime number is 7, since it can only be formed by multiplying the numbers 1 and 7. Other examples include 2, 3, 5, 11, etc. Numbers that can be formed with two other natural numbers, that are greater than 1, are called composite numbers. Examples of this include numbers like, 4, 6, 9, etc. Prime numbers are widely used in number theory due to the fundamental theorem of arithmetic. This theorem states that natural numbers greater than 1 are either prime, or can be factored as a product of prime numbers. As an example, the number 60 can be factored into a product of prime numbers as follows:. Prime factorization is the decomposition of a composite number into a product of prime numbers. There are many factoring algorithms, some more complicated than others.

Hospice izle

Solution: When we divide by it leaves a remainder. Study now See answer 1. The factors of in pairs are:. Factors of 25 - The factors of 25 are 1, 5, Therefore, the number is not a factor of Hence, [1, 3, 9] are the common factors of and All Rights Reserved. Work out as a product of primes? What is prime factorization of ? Maths Formulas. Repeat Steps 1 and 2, using as the new focus.

Factors of are numbers that, when multiplied in pairs give the product as There are overall 18 factors of among which is the biggest factor and 3, 5, 7 are its prime factors.

Further dividing by 3 gives a non-zero remainder. Maths Formulas. Log in. Here are the steps for finding the prime factorization of Step 1: Find the smallest prime number that is larger than 1, and is a factor of The factors of are 1, 3, 5, 7, 9, 15, 21, 35, 45, 49, 63, , , , , , , and factors of are 1, 17, 73, Solution: When we divide by it leaves a remainder. These factors are either prime numbers or composite numbers. Math worksheets and visual curriculum. Commercial Maths. Q: Express as the product of prime factors off ? We stop ultimately if the next prime factor doesn't exist or when we can't divide any further. In other words, finding the factors of is like breaking down the number into all the smaller pieces that can be used in a multiplication problem to equal Kindergarten Worksheets. What number does 7 5 and 9 go into?