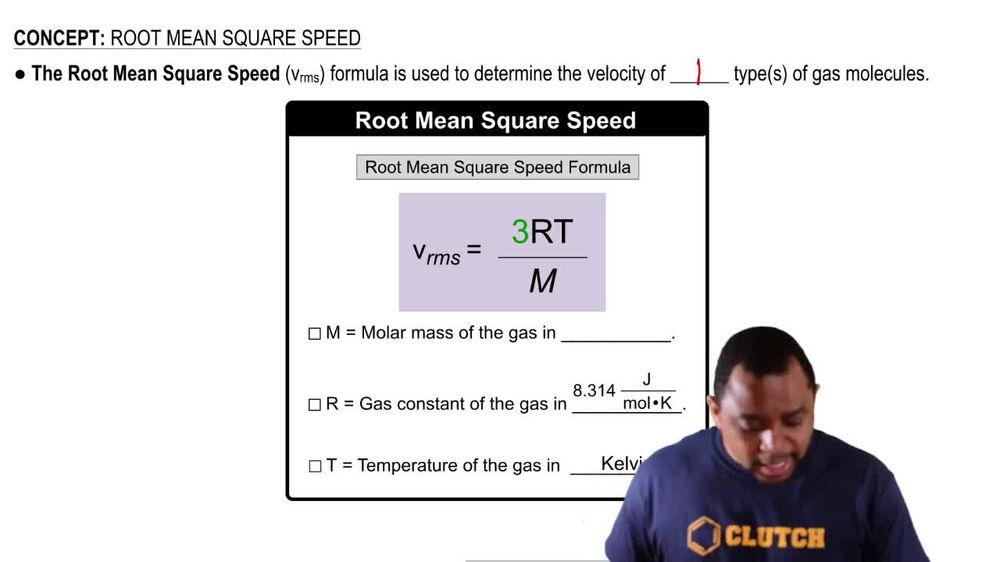
Root mean square speed

We have examined pressure and temperature based on their macroscopic definitions.

Determine the most probable, average and root-mean-square speed of gas molecules described by the Maxwell-Boltzmann distribution. Maxwell-Boltzmann distribution describes a classical system of distinguishable particles, such as for example molecules. A distribution function for the magnitude of velocity of the molecules is defined as follows. The most probable speed of gas molecules described by the Maxwell-Boltzmann distribution is the speed at which distribution graph reaches its maximum. Thus, if we know the formula of this distribution, we just need to differentiate it and consider the derivative to be equal to zero.

Root mean square speed

Other sections state that increasing the temperature increases the speeds at which molecules move. We are now in a position to find just how large that increase is for a gaseous substance. Combining the ideal gas law with Eq. Since N is the number of molecules and m is the mass of each molecule, Nm is the total mass of gas. The rms velocity is directly proportional to the square root of temperature and inversely proportional to the square root of molar mass. Thus quadrupling the temperature of a given gas doubles the rms velocity of the molecules. Doubling this average velocity doubles the number of collisions between gas molecules and the walls of a container. It also doubles the impulse of each collision. Thus the pressure quadruples. The inverse proportionality between root-mean-square velocity and the square root of molar mass means that the heavier a molecule is, the slower it moves, which is verified by the examples below. We can compare the rates of effusion or diffusion of a known gas with that of an unknown gas to determine the molar mass of the unknown gas.

Square root of the mean square.

This example problem demonstrates how to calculate the root mean square RMS velocity of particles in an ideal gas. This value is the square root of the average velocity-squared of molecules in a gas. While the value is an approximation, especially for real gases, it offers useful information when studying kinetic theory. What is the average velocity or root mean square velocity of a molecule in a sample of oxygen at 0 degrees Celsius? Gases consist of atoms or molecules that move at different speeds in random directions. The root mean square velocity RMS velocity is a way to find a single velocity value for the particles.

The gas laws that we have seen to this point, as well as the ideal gas equation, are empirical, that is, they have been derived from experimental observations. The mathematical forms of these laws closely describe the macroscopic behavior of most gases at pressures less than about 1 or 2 atm. Although the gas laws describe relationships that have been verified by many experiments, they do not tell us why gases follow these relationships. The kinetic molecular theory KMT is a simple microscopic model that effectively explains the gas laws described in previous modules of this chapter. This theory is based on the following five postulates described here. The test of the KMT and its postulates is its ability to explain and describe the behavior of a gas. The various gas laws can be derived from the assumptions of the KMT, which have led chemists to believe that the assumptions of the theory accurately represent the properties of gas molecules. Recalling that gas pressure is exerted by rapidly moving gas molecules and depends directly on the number of molecules hitting a unit area of the wall per unit of time, we see that the KMT conceptually explains the behavior of a gas as follows:. The previous discussion showed that the KMT qualitatively explains the behaviors described by the various gas laws. The postulates of this theory may be applied in a more quantitative fashion to derive these individual laws.

Root mean square speed

The laws that describe the behavior of gases were well established long before anyone had developed a coherent model of the properties of gases. In this section, we introduce a theory that describes why gases behave the way they do. The theory we introduce can also be used to derive laws such as the ideal gas law from fundamental principles and the properties of individual particles.

Joints traduccion

You may accept or manage your choices by clicking below, including your right to object where legitimate interest is used, or at any time in the privacy policy page. We can now give an equation for the internal energy of a monatomic ideal gas. Speed for which the derivate equals zero is the most probable speed. We now use the definition of the average, which we denote with a bar, to find the force:. The average translational kinetic energy depends only on absolute temperature. Because the right-hand side is the same for any gas at a given temperature in a container of a given volume, the left-hand side is the same as well. However, this is not true for an arbitrary waveform, which may not be periodic or continuous. Topics Typically Covered in Grade 11 Chemistry. For example, for either a triangular or sawtooth wave:. This article needs additional citations for verification. Create profiles for personalised advertising. The table of contents will list only tasks having one of the required ranks in corresponding rankings and at least one of the required tags overall.

For alternating electric current , RMS is equal to the value of the constant direct current that would produce the same power dissipation in a resistive load. The RMS value of a set of values or a continuous-time waveform is the square root of the arithmetic mean of the squares of the values, or the square of the function that defines the continuous waveform. In physics, the RMS current value can also be defined as the "value of the direct current that dissipates the same power in a resistor.

Use limited data to select advertising. He holds bachelor's degrees in both physics and mathematics. We can hardly compare this result with our intuition about gas molecules, but it gives us a picture of molecules colliding with extremely high frequency. In fact, the light is coming from the Sun. Cyberphysics - a web-based teaching aid - for students of physics, their teachers and parents Identify the knowns and unknowns and determine which equations to use to solve the problem. In fact, we will take two averages: one over time to get the average force exerted by one molecule with a given velocity, and then another average over molecules with different velocities. Kinetic molecular theory tries to explain the properties of gases by investigating the behavior of individual atoms or molecules making up the gas. The following figure shows the Maxwell-Boltzmann distribution of the magnitude of velocity of gas molecules. Machine learning evaluation metrics.