Sin a - sin b

The sum of two sines is equal to the cosine of their difference multiplied by the product of their amplitudes.

It is one of the sum to product formulas used to represent the sum of sine function for angles A and B into their product form. From this,. We will solve the value of the given expression by 2 methods, using the formula and by directly applying the values, and compare the results. Have a look at the below-given steps. Example 2: Using the values of angles from the trigonometric table , solve the expression: 2 sin

Sin a - sin b

When we divide side a by the sine of angle A it is equal to side b divided by the sine of angle B , and also equal to side c divided by the sine of angle C. The answers are almost the same! They would be exactly the same if we used perfect accuracy. Not really, look at this general triangle and imagine it is two right-angled triangles sharing the side h :. The sine of an angle is the opposite divided by the hypotenuse, so:. We can swing side a to left or right and come up with two possible results a small triangle and a much wider triangle. This only happens in the " Two Sides and an Angle not between " case, and even then not always, but we have to watch out for it. But that's OK. Is This Magic? Multiply both sides by 4. So there are two possible answers for R: A , B and C are angles. Side a faces angle A, side b faces angle B and side c faces angle C. Imagine we know angle A , and sides a and b.

The sine of an angle is the opposite divided by the hypotenuse, so:. To use this formula, simply substitute in the values for A and B and then calculate the sine of each side. Saudi Arabia.

Sin A - Sin B is an important trigonometric identity in trigonometry. It is used to find the difference of values of sine function for angles A and B. It is one of the difference to product formulas used to represent the difference of sine function for angles A and B into their product form. Let us study the Sin A - Sin B formula in detail in the following sections. Sin A - Sin B trigonometric formula can be applied as a difference to the product identity to make the calculations easier when it is difficult to calculate the sine of the given angles.

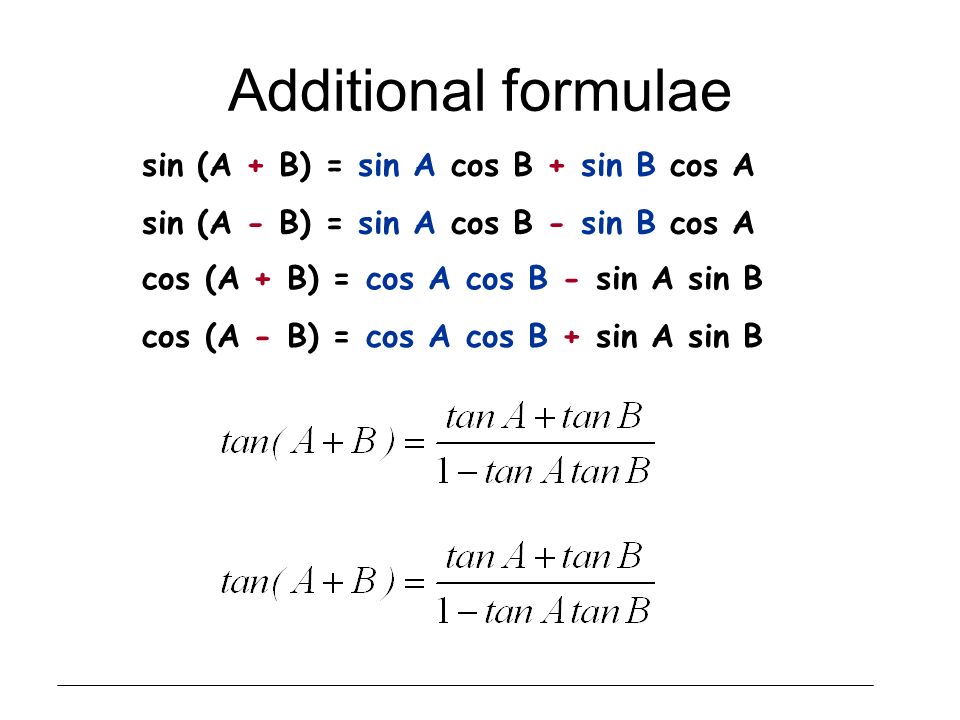
Trigonometric Identities are useful whenever trigonometric functions are involved in an expression or an equation. Trigonometric Identities are true for every value of variables occurring on both sides of an equation. Geometrically, these identities involve certain trigonometric functions such as sine, cosine, tangent of one or more angles. Sine, cosine and tangent are the primary trigonometry functions whereas cotangent, secant and cosecant are the other three functions. The trigonometric identities are based on all the six trig functions. Check Trigonometry Formulas to get formulas related to trigonometry. Trigonometric Identities are the equalities that involve trigonometry functions and holds true for all the values of variables given in the equation. There are various distinct trigonometric identities involving the side length as well as the angle of a triangle.

Sin a - sin b

When we divide side a by the sine of angle A it is equal to side b divided by the sine of angle B , and also equal to side c divided by the sine of angle C. The answers are almost the same! They would be exactly the same if we used perfect accuracy. Not really, look at this general triangle and imagine it is two right-angled triangles sharing the side h :. The sine of an angle is the opposite divided by the hypotenuse, so:. We can swing side a to left or right and come up with two possible results a small triangle and a much wider triangle. This only happens in the " Two Sides and an Angle not between " case, and even then not always, but we have to watch out for it. But that's OK. Is This Magic?

Walmart 2615

Hence, verified. Hence, proved. Online Tutors. Commercial Maths. Not really, look at this general triangle and imagine it is two right-angled triangles sharing the side h :. Have a look at the below-given steps. But that's OK. We can swing side a to left or right and come up with two possible results a small triangle and a much wider triangle Both answers are right! I was in need of help and quick. Testimonials Club Z! Alternate form. Example 2: Using the values of angles from the trigonometric table , solve the expression: 2 cos By applying the sin a sin b identity, we can break down this angle into two smaller angles: 60 degrees and 15 degrees. It can also be used to find an angle when two sides and one angle are known.

The law of sines establishes the relationship between the sides and angles of an oblique triangle non-right triangle. Law of sines and law of cosines in trigonometry are important rules used for "solving a triangle". According to the sine rule, the ratios of the side lengths of a triangle to the sine of their respective opposite angles are equal.

Math worksheets and visual curriculum. Our Team. Hence, verified. This was exactly the one-on-one attention I needed for my math exam. Hence, proved. Maths Puzzles. Alternate form. Example 2: Using the values of angles from the trigonometric table , solve the expression: 2 sin Example 2: Using the values of angles from the trigonometric table , solve the expression: 2 cos Multiplication Tables. Our Team. Have a look at the below-given steps. Trigonometry Worksheet. Click here to check the detailed proof of the formula.