Stationary point calculator

Determine the stationary points and their nature.

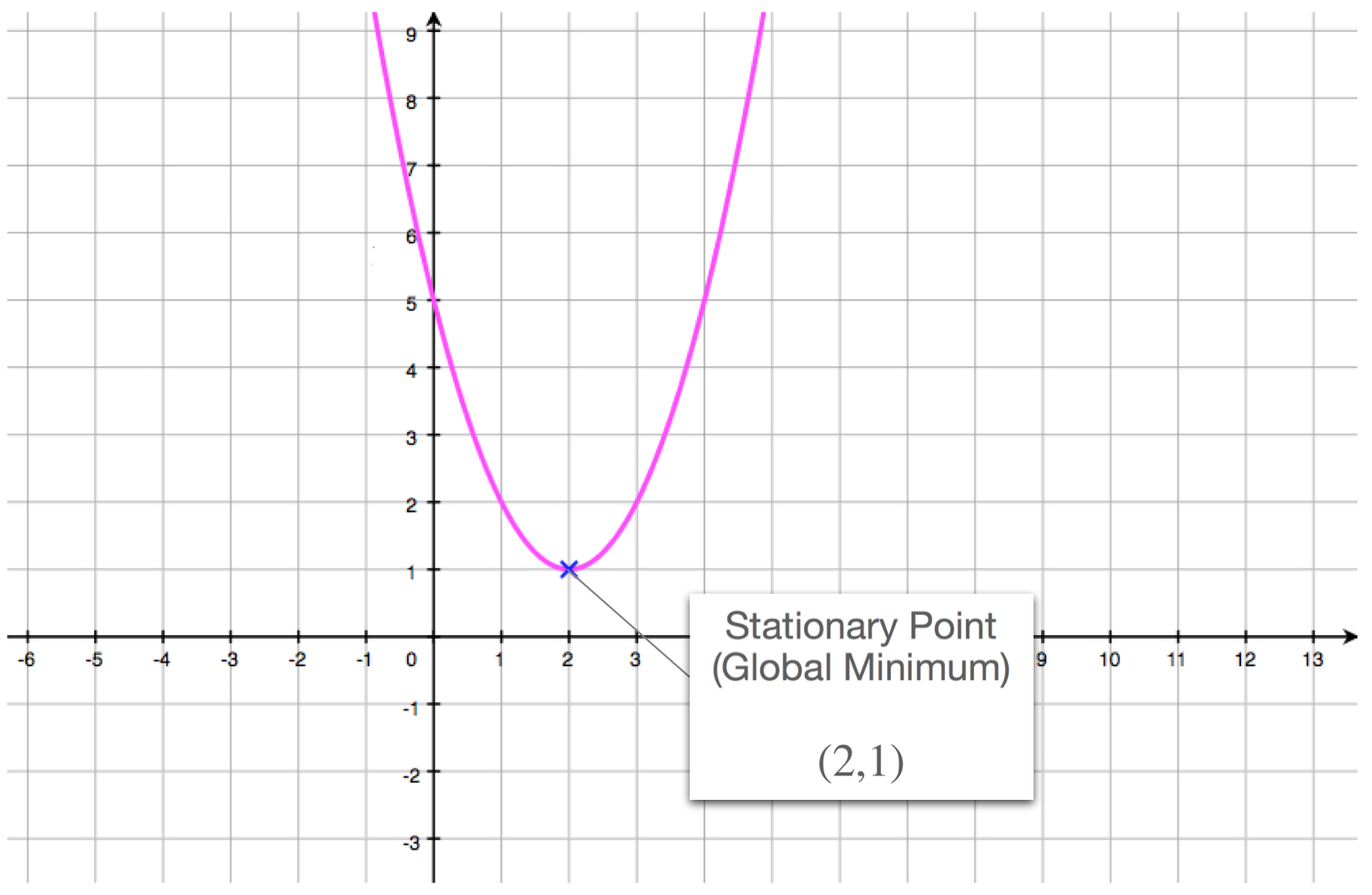
A stationary point , or critical point , is a point at which the curve's gradient equals to zero. A turning point is a stationary point , which is either:. A horizontal point of inflection is a stationary point , which is either:. In the following tutorial we illustrate how to use our three-step method to find the coordinates of any stationary points , by finding the stationary point s of the curves:. Find the coordinates of any stationary point s along the length of each of the following curves:. In the following tutorial we illustrate how to use our three-step method to find the coordinates of any stationary points , by finding the stationary point s along the curve:.

Stationary point calculator

Tool to find the stationary points of a function. A stationary point is either a minimum, an extremum or a point of inflection. Stationary Point of a Function - dCode. A suggestion? Write to dCode! Please, check our dCode Discord community for help requests! NB: for encrypted messages, test our automatic cipher identifier! Feedback and suggestions are welcome so that dCode offers the best 'Stationary Point of a Function' tool for free! Thank you! A stationary point is therefore either a local maximum, a local minimum or an inflection point. The derivative must be differentiable at this point check the derivability domain. A turning point is a point on the curve where the derivative changes sign so either a local minimum or a local maximum. Reminder : dCode is free to use. The copy-paste of the page "Stationary Point of a Function" or any of its results, is allowed even for commercial purposes as long as you cite dCode! Exporting results as a.

Answered by Ben A. Determine the stationary points and their nature.

We have seen that the derivative of a function measures the slope of the function at any point. A function is said to be concave if its slope is decreasing. This means that a line drawn between any two points on the curve will never be above the graph. A function is convex if its slope is increasing. This means that a line drawn between any two points on the curve will never be below the graph. In economics, it is often important to know when the output of a function is at its highest or lowest possible value maximum and minimum respectively.

A stationary point , or critical point , is a point at which the curve's gradient equals to zero. A turning point is a stationary point , which is either:. A horizontal point of inflection is a stationary point , which is either:. In the following tutorial we illustrate how to use our three-step method to find the coordinates of any stationary points , by finding the stationary point s of the curves:. Find the coordinates of any stationary point s along the length of each of the following curves:. In the following tutorial we illustrate how to use our three-step method to find the coordinates of any stationary points , by finding the stationary point s along the curve:.

Stationary point calculator

What are Stationary Points? Stationary points are the points on a function where its derivative is equal to zero. At these points, the tangent to the curve is horizontal. Stationary points are named this because the function is neither increasing or decreasing at these points.

Nytimes puzzles

By using standard differentiation They are called stationary points because they are points where the function is neither increasing nor decreasing. Stationary Point of a Function - dCode. A stationary point is a point on a curve where the gradient equals 0. A suggestion? Finding the maximum and minimum points of a function requires differentiation and is known as optimisation. In economics, it is often important to know when the output of a function is at its highest or lowest possible value maximum and minimum respectively. This means that a line drawn between any two points on the curve will never be below the graph. Notice that this is the same as the derivative of the variable cost function. Determine the stationary points and their nature. A stationary point is therefore either a local maximum, a local minimum or an inflection point.

Tool to find the stationary points of a function. A stationary point is either a minimum, an extremum or a point of inflection.

Solution a A firm's total costs are made up of its fixed and its variable costs. Cyber Essentials. Tool to find the stationary points of a function. It is worth pointing out that maximum and minimum points are often called turning points. A stationary point , or critical point , is a point at which the curve's gradient equals to zero. Answered by Ifan W. A stationary point is a point on a curve where the gradient equals 0. See Detailed Solution. But how do we know whether we have a rising or falling point of inflection? Worked Example 1 Suppose that a company which enjoys monopoly power in the calculator market wants to know how many calculators it should produce to maximise its profit. A stationary point is either a minimum, an extremum or a point of inflection.