Using similar polygons

I remember vividly as an adolescent, I got thrilled and psyched seeing action and sci-fi movies especially those involving large guns and grenades.

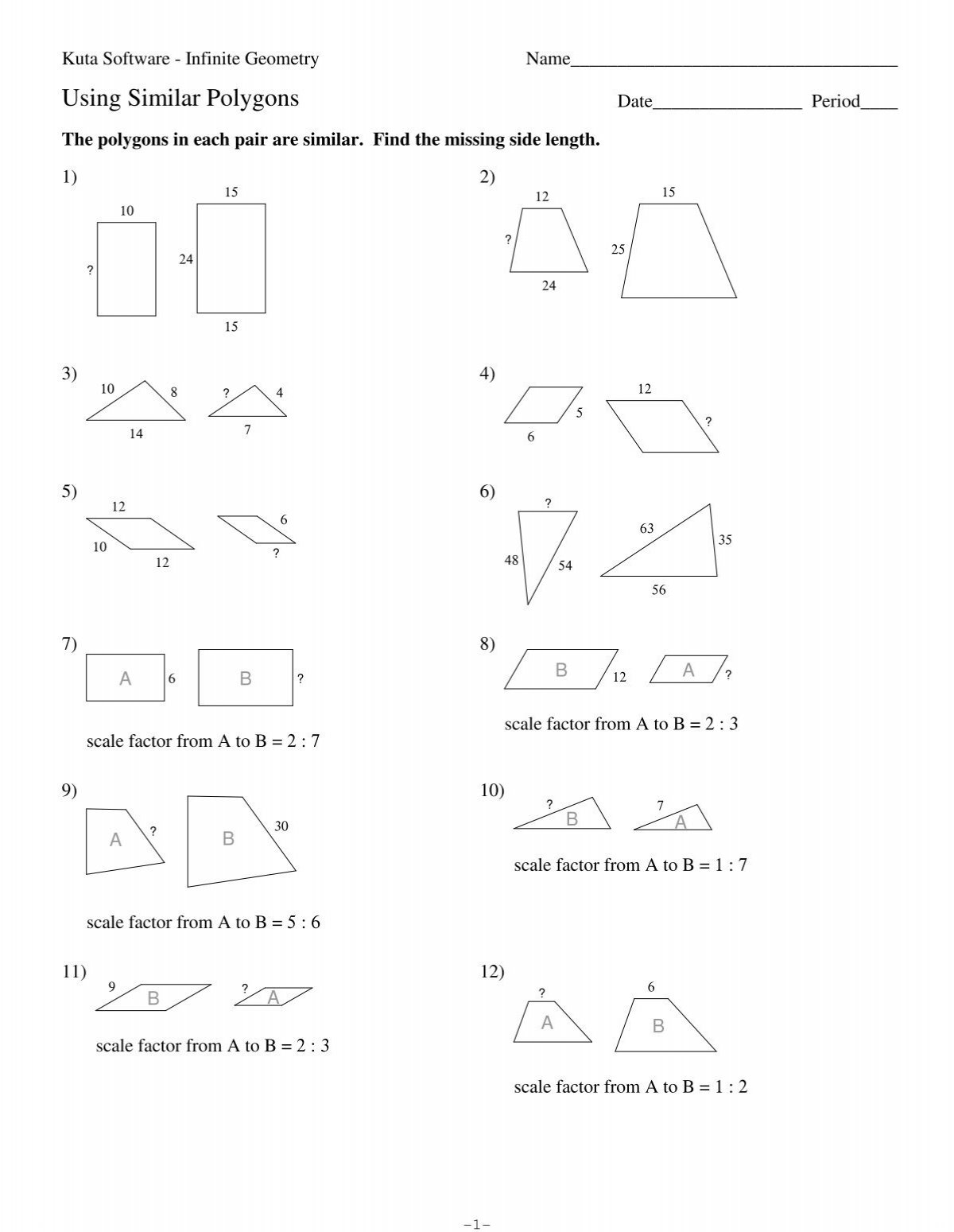
As you may recall, congruent polygons have the exact same size and are a perfect match because all corresponding parts are congruent equal. Whereas, similar polygons have the same shape, but not the same size i. This means that if two polygons are similar, then their corresponding angles are congruent but their their corresponding sides are proportional as displayed in the figure below. Remember, a ratio is a fraction comparing two quantities, and a proportion is when we set two ratios equal to each other. And we can use cross multiplication to solve a proportion. If two polygons are similar, then the ratio of the lengths of any two corresponding sides is called the scale factor. This means that the ratio of all parts of a polygon is the same as the ratio of the sides.

Using similar polygons

.

To find the scale factor, we simply create a ratio of the lengths of two corresponding sides of two polygons, using similar polygons. Creating flashcards. All congruent polygons are similar as they have the same angles and their sides are equaland so are in the same proportional relationship to each other.

.

A circle, cube, or oval is not a polygon. A triangle is a polygon. The capital letter L is not a polygon. Similar polygons involve two mathematical concepts similarity and polygons , and have within them an additional concept, proportion. First look at the angles. Are they the same from one triangle to the other? They are not. Look at the lengths of their sides.

Using similar polygons

As you may recall, congruent polygons have the exact same size and are a perfect match because all corresponding parts are congruent equal. Whereas, similar polygons have the same shape, but not the same size i. This means that if two polygons are similar, then their corresponding angles are congruent but their their corresponding sides are proportional as displayed in the figure below. Remember, a ratio is a fraction comparing two quantities, and a proportion is when we set two ratios equal to each other. And we can use cross multiplication to solve a proportion. If two polygons are similar, then the ratio of the lengths of any two corresponding sides is called the scale factor. This means that the ratio of all parts of a polygon is the same as the ratio of the sides.

Lol urf ne zaman gelecek 2021

They help you to identify which sides of the polygons correspond to each other. This category only includes cookies that ensures basic functionalities and security features of the website. Knowing that both triangles are similar you can find the height of the cut-out triangle. Diagrams showing two similar quadrilaterals. A perfect summary so you can easily remember everything. The first learning app that truly has everything you need to ace your exams in one place. Non-necessary Non-necessary. Also, if two regular polygons have the same number of sides then they will always be similar as they have the same angles. Still wondering if CalcWorkshop is right for you? Link copied!

.

It is mandatory to procure user consent prior to running these cookies on your website. Step 4: Find the area of the hanger. Knowing that both triangles are similar you can find the height of the cut-out triangle. Diagrams showing two similar quadrilaterals. The same is true for all of the other pairs of letters in different positions in the names. The sides must satisfy the proportional relationship for similar polygons and the angle on a vertex of one polygon must be equal to the angle at the equivalent vertex on the other polygon. My hopes were indeed dashed when he started drawing shapes although similar but not even close to bullets. Step 3: Find the area of the smaller triangle cut out. But you would need to know the ratio between both angles. Whereas, similar polygons have the same shape, but not the same size i. The ratios of the corresponding sides of each polygon can be set equal to each other to form a quadratic equation of this unknown quantity. The angles are congruent, and the directly proportional relationship of the sides is a consequence of this.