Altitude of equilateral triangle formula

The equilateral triangle calculator will help you with calculations of standard triangle parameters. Whether you are looking for the equilateral triangle area, its height, perimeter, circumradius, or inradius, this great tool is a safe bet.

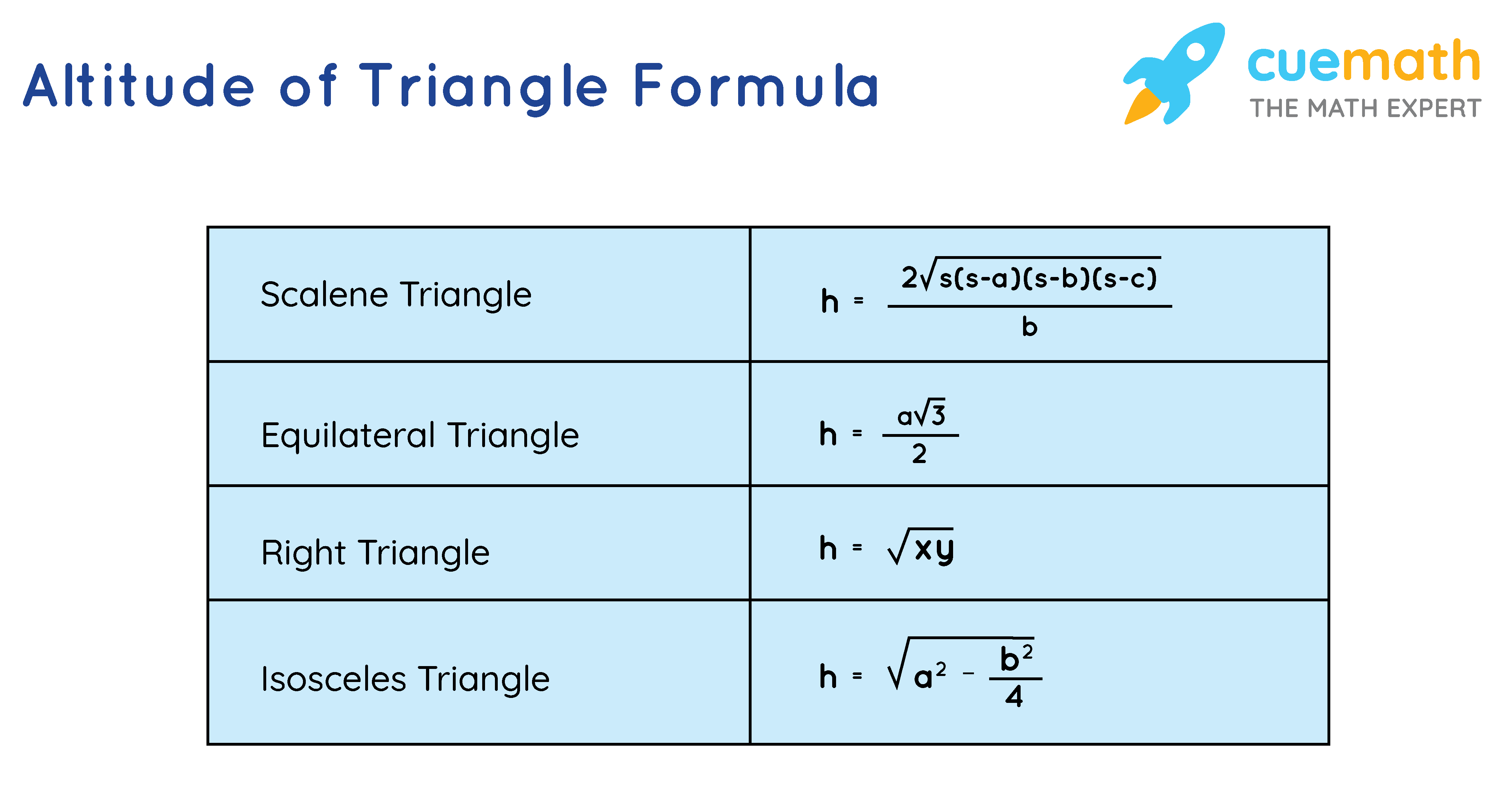
The perpendicular drawn from the vertex to the opposite side of the triangle is called the altitude of a triangle. The altitude of a triangle formula gives us the height of the triangle. The altitude of a triangle formula is interpreted and different formulas are given for different types of triangles. The altitude is used for the calculation of the area of a triangle. The altitude of a triangle formula can be expressed as follows.

Altitude of equilateral triangle formula

The internal angles of any given equilateral triangle are of the same measure, that is, equal to 60 degrees. Triangles are classified into three sorts based on the length of their sides:. Scalene triangle: The sides and the angles of the scalene triangle are not equal. Isosceles triangle: An isosceles triangle has two equal sides and two equal angles. Equilateral triangle: All sides and angles of the equilateral triangle are equal. The region enclosed by the three sides of an equilateral triangle is defined as the area of the equilateral triangle. It is expressed in square units. The common units used to express the area of an equilateral triangle are in 2 , m 2 , cm 2 and yd 2. Below the area of the equilateral triangle formula, the altitude of the equilateral triangle formula, the perimeter of the equilateral triangle formula, and the semi-perimeter of an equilateral triangle are discussed. The area of an equilateral triangle is the amount of space that it occupies in a 2-dimensional plane. The area occupied between the sides of an equilateral triangle in a plane is calculated using the equilateral triangle area formula. The formula for calculating the area of a triangle with a known base and height is:.

Perimeter P is known; find a, s, K, and h. Let us understand this with an example. According to the definition of equilateral triangles, all internal angles are equal.

The height of an equilateral triangle is a straight line that is drawn from the vertex to the opposite side of the triangle in such a way that it divides the triangle into two equal right-angled triangles. This is also known as the altitude of the triangle which starts from the vertex and is the perpendicular bisector of the opposite side. An equilateral triangle is a triangle in which all the sides are of equal length and all the angles are of equal measure. Let us learn more about the height of equilateral triangle in this article. The height of an equilateral triangle is a line that is drawn from any vertex of the triangle on the opposite side. This line is the perpendicular bisector of the opposite side. The height of an equilateral triangle is also known as the altitude which divides the triangle into two congruent right-angled triangles as shown in the following figure.

The height of an equilateral triangle is a straight line that is drawn from the vertex to the opposite side of the triangle in such a way that it divides the triangle into two equal right-angled triangles. This is also known as the altitude of the triangle which starts from the vertex and is the perpendicular bisector of the opposite side. An equilateral triangle is a triangle in which all the sides are of equal length and all the angles are of equal measure. Let us learn more about the height of equilateral triangle in this article. The height of an equilateral triangle is a line that is drawn from any vertex of the triangle on the opposite side. This line is the perpendicular bisector of the opposite side. The height of an equilateral triangle is also known as the altitude which divides the triangle into two congruent right-angled triangles as shown in the following figure. An Equilateral triangle is defined as a triangle where all three sides and angles are equal.

Altitude of equilateral triangle formula

The altitude of a triangle is a perpendicular that is drawn from the vertex of a triangle to the opposite side. Since there are three sides in a triangle, three altitudes can be drawn in it. Different triangles have different kinds of altitudes. The altitude of a triangle which is also called its height is used in calculating the area of a triangle and is denoted by the letter 'h'. The altitude of a triangle is the perpendicular line segment drawn from the vertex of the triangle to the side opposite to it.

Thesaurus for full

An equilateral triangle is a triangle in which all the sides are of equal length and all the angles are of equal measure. The common units used to express the area of an equilateral triangle are in 2 , m 2 , cm 2 and yd 2. We know that all the sides of an equilateral triangle are equal and the altitude divides the triangle into two congruent right-angled triangles. Example: If one side of an equilateral triangle is 12 units, what is its height? The perimeter of a triangle is equal to the sum of the length of its three sides, whether they are equal or not. Using the Pythagoras theorem, the formula for the height of the equilateral can be derived and expressed as follows,. How to find the altitude of an equilateral triangle given the perimeter? Maths Questions. This means if the side length of an equilateral triangle is known, then the altitude can be easily calculated using this formula. This regular triangle has all sides equal, so the formula for the perimeter is:. Already booked a tutor? Height of Equilateral Triangle The height of an equilateral triangle is a straight line that is drawn from the vertex to the opposite side of the triangle in such a way that it divides the triangle into two equal right-angled triangles. Save Article Save. Free fall Calculator Our free fall calculator can find the velocity of a falling object and the height it drops from.

The altitude of a triangle is the perpendicular line segment drawn from the vertex to the opposite side of the triangle. It may lie inside or outside the triangle, based on the types of triangles. The altitude of a triangle basically defines the height, when we have to measure the area of a triangle, with respect to the base.

The perimeter of a square is referred to as the total length of the boundary enclosing the geometrical figure. How to find the length of an equilateral triangle when the perimeter is given? Using the Pythagoras theorem, the formula for the height of the equilateral can be derived and expressed as follows,. To find the area of an equilateral triangle, follow the given instructions: Take the square root of 3 and divide it by 4. This is also known as the altitude of the triangle which starts from the vertex and is the perpendicular bisector of the opposite side. To find the height of an equilateral triangle, proceed as follows: Take the square root of 3 and divide it by 2. About Us. Our Team. What is 2i equal to? The height of an equilateral can be easily calculated if the side is given. The altitude is used for the calculation of the area of a triangle. Next Fraud Prevention and Privacy Laws. How to find the ratio in which a point divides a line?