Khan academy finite element method

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos.

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos. Modeling situations with differential equations. About About this video Transcript. Differential equations are equations that relate a function with one or more of its derivatives. This means their solution is a function!

Khan academy finite element method

Personalise your OpenLearn profile, save your favourite content and get recognition for your learning. Start this free course now. Just create an account and sign in. Enrol and complete the course for a free statement of participation or digital badge if available. The basic principles underlying the FEM are relatively simple. Consider a body or engineering component through which the distribution of a field variable, e. Examples could be a component under load, temperatures subject to a heat input, etc. The body, i. The elements are assumed to be connected to one another, but only at interconnected joints, known as nodes. It is important to note that the elements are notionally small regions, not separate entities like bricks, and there are no cracks or surfaces between them. There are systems available that do model materials and structures comprising actual discrete elements such as real masonry bricks, particle mixes, grains of sand, etc. The complete set, or assemblage of elements, is known as a mesh. The process of representing a component as an assemblage of finite elements, known as discretisation, is the first of many key steps in understanding the FEM of analysis. An example is illustrated in Figure 1. This is a plate-type component modelled with a number of mostly rectangular ish elements with a uniform thickness into the page or screen that could be, say, 2 mm.

Log in. Well we have just proven it. Sort by: Top Voted.

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos. About About this video Transcript. Proving an expression for the sum of all positive integers up to and including n by induction. Created by Sal Khan.

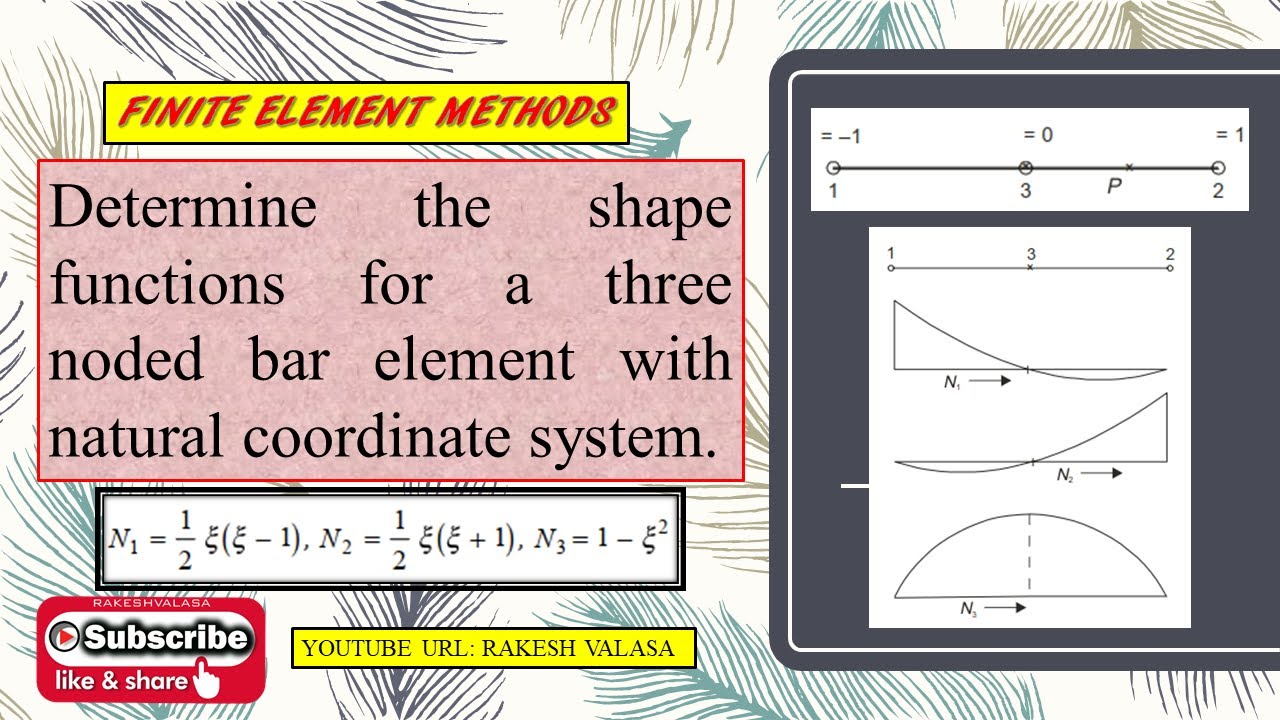
Forgot password? New user? Sign up. Existing user? Log in. Already have an account? Log in here. The finite element method FEM is a numerical method for solving partial differential equations PDE that occur in problems of engineering and mathematical physics. The basic concept of FEM is to divide continuous bodies into a mesh of simple parts, the so-called finite elements.

Khan academy finite element method

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos. Equivalent systems of equations and the elimination method. About About this video Transcript.

Hd todaytv

Times k plus 1 plus 1. Khan Academy covers only some very basic parts of differential equations. Our base case is going to be 1. So let's work through this together. So a traditional equation, maybe I shouldn't say traditional equation, differential equations have been around for a while. And the reason why this is all you have to do to prove this for all positive integers it's just imagine: Let's think about all of the positive integers right over here. And I encourage you to pause the video again and verify that it's a solution. Now, we can rewrite this. Search for courses, skills, and videos. We use k to reduce the confusion between the two and k resembles a constant which you may use in calculus math. There is no other positive integer up to and including 1.

The finite element method FEM is a powerful technique originally developed for numerical solution of complex problems in structural mechanics , and it remains the method of choice for complex systems. In the FEM, the structural system is modeled by a set of appropriate finite elements interconnected at discrete points called nodes.

Posted 7 months ago. And you might have just caught from how I described it that the solution to a differential equation is a function, or a class of functions. Now what I want to do in this video is prove to you that I can write this as a function of N, that the sum of all positive integers up to and including N is equal to n times n plus one, all of that over 2. Sort by: Top Voted. All it specifies is that there will always be three different roots. Subspaces and the basis for a subspace : Vectors and spaces Vector dot and cross products : Vectors and spaces Matrices for solving systems by elimination : Vectors and spaces Null space and column space : Vectors and spaces. We have a common denominator of 2 and I'll write this in a different colour here. Posted 7 years ago. The result is a triangle:. And we are done. Posted 4 years ago. Here 2x is an angle then why there is a need of differentiating the angle 2x. So the second derivative is going to be e to the x plus two times e to the x is indeed going to be equal to three times e to the x. For now let's just think about or at least look at what a differential equation actually is. Proving an expression for the sum of all positive integers up to and including n by induction.