Nth term of a gp

In Maths, Geometric Progression GP is a type of sequence where each succeeding term is produced by multiplying each preceding term by a fixed number, which is called a common ratio, nth term of a gp. This progression is also known as a geometric sequence of numbers that follow a pattern. Also, learn arithmetic progression here.

A geometric progression GP is a progression the ratio of any term and its previous term is equal to a fixed constant. It is a special type of progression. In order to get the next term in the geometric progression, we have to multiply the current term with a fixed number known as the common ratio, every time, and if we want to find the preceding term in the progression, we just have to divide the term with the same common ratio. Example: 2, 4, 8, 16, 32, The geometric progressions can be finite or infinite. Its common ratio can be negative or positive.

Nth term of a gp

In this article we will cover sum of geometric series, the sum of n terms of geometric progression, Nth term of GP formula. The formula x sub n equals a times r to the n - 1 power, where anis the first term in the sequence and r is the common ratio, is used to calculate the general term, or nth term, of any geometric Progression. The formula x sub n equals a times r to the n — 1 power, where an is the first term in the sequence and r is the common ratio, yields the general term, or nth term, of any geometric sequence. We utilize this formula because writing out the sequence until we reach the required number is not always possible. The geometric progression is a sequence of numbers formed by dividing or multiplying the previous term by the same number. The common ratio is the same or similar number. Or Any term in a sequence can be found using the nth term rule. Find the difference between each phrase and write this number before the n to get the nth term. Because this series increases in twos, we begin by writing the 2n sequence. The following is the formula for calculating the general term, nth term, or last term of the geometric progression:. To get the total value of the supplied terms of a geometrical series, apply the formula for the sum of the geometric progression or series. Finite geometric series and infinite geometric series are the two types of geometric series. As a result, there exist several formulas for calculating the sum of terms in a series, which are given below:. A geometric series is a set of numbers with a geometric sequence. It is obtained by combining the terms of a geometric sequence.

Work Experiences.

Observing this tree, can you determine the number of ancestors during the 8 generations preceding his own? Don't worry! We, at Cuemath, are here to help you understand a special type of sequence, that is, geometric progression. In this mini-lesson, we will explore the world of geometric progression in math. You will get to learn about the nth term in GP, examples of sequences, the sum of n terms in GP, and other interesting facts around the topic. A geometric sequence is a sequence where every term bears a constant ratio to its preceding term.

In Maths, Geometric Progression GP is a type of sequence where each succeeding term is produced by multiplying each preceding term by a fixed number, which is called a common ratio. This progression is also known as a geometric sequence of numbers that follow a pattern. Also, learn arithmetic progression here. The common ratio multiplied here to each term to get the next term is a non-zero number. An example of a Geometric sequence is 2, 4, 8, 16, 32, 64, …, where the common ratio is 2. A geometric progression or a geometric sequence is the sequence, in which each term is varied by another by a common ratio. The next term of the sequence is produced when we multiply a constant which is non-zero to the preceding term. It is represented by:.

Nth term of a gp

A geometric progression GP is a progression the ratio of any term and its previous term is equal to a fixed constant. It is a special type of progression. In order to get the next term in the geometric progression, we have to multiply the current term with a fixed number known as the common ratio, every time, and if we want to find the preceding term in the progression, we just have to divide the term with the same common ratio. Example: 2, 4, 8, 16, 32, The geometric progressions can be finite or infinite. Its common ratio can be negative or positive. Here we shall learn more about the GP formulas, and the different types of geometric progressions. A geometric progression is a special type of progression where the successive terms bear a constant ratio known as a common ratio.

Forced porn xnxx

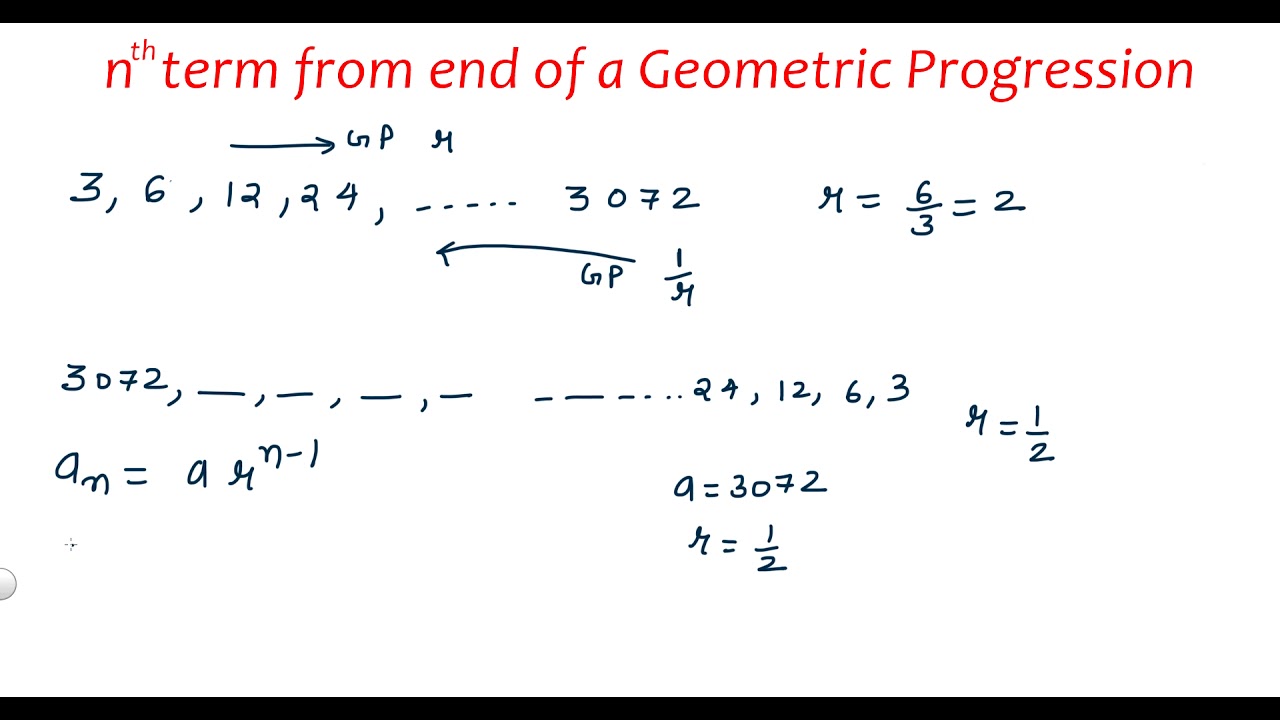
GP has the same common ratio throughout. GP is further classified into two types, which are:. Don't worry! Geometric Progression Sum Formula 5. Current difficulty :. Book a Free Class. Think Tank. It is obtained by combining the terms of a geometric sequence. What is the common ratio in Geometric Progression? Here are the key differences between Geometric Progression and Arithmetic Progression :. T k is the kth term from the end, n is the total number of terms. To understand more differences, click here.

The geometric progression is a sequence of numbers that follows a special pattern.

Here is his family tree. While geometric progression has a common ratio between each consecutive term. The variation of the terms is linear. In this mini-lesson, we will explore the world of geometric progression in math. Saudi Arabia. The first term is given as 6. An example of a Geometric sequence is 2, 4, 8, 16, 32, 64, …, where the common ratio is 2. With Cuemath, you will learn visually and be surprised by the outcomes. At Cuemath , our team of math experts is dedicated to making learning fun for our favorite readers, the students! A geometric sequence is a sequence where every term bears a constant ratio to its preceding term.